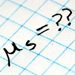Experiments in support of engineering.
Theory is important, but sometimes the most efficient and accurate way to solve an engineering problem is through testing and experimentation.
Perhaps the best example of this is friction. Pretty simple right? You've got two materials sliding on each other; the friction force is related to the normal force by the coefficient of friction. Just look up the coefficient in your favourite handbook, or punch in into Google, and you're good to go, right? Wrong. Well, okay, if you're designing something with steel on steel friction, clean and dry with an excellent surface finish, you'll probably be okay. Otherwise it is basically a shot in the dark. Let's say one of your materials is a 30A durometer urethane, and the other is hard anodized aluminum. Now, take an hour and see if you can find a coefficient for that pair. Got something? Didn't think so... What you may have found are some numbers for urethanes of unspecified durometer against steel. Or even better, against an unspecified material! You might also have found numbers from different sources that disagree substantially. Rarely is there any detailed information on the conditions under which the coefficient was determined-what exactly do they mean by ‘oxidized'? Lubricated? Is this a sterile lab environment, or on the dirty shop floor?
Sometimes it is good enough to just design around the worst case number, and throw a factor of safety at it. Other times, that's just not good enough. Here's a good example I had to deal with recently: I was designing a tool that needed to pivot easily about an axis, and clamp in position. I had a limited number of materials to work with for the shaft, bushings, and clamp element-basically stainless steels and aluminum bronze. I had a fairly broad range of coefficient values to choose from that represented similar materials. If I chose a value on the low end of the range, I just couldn't get enough clamping force to prevent the tool from rotating. If I chose a value on the high end of the range, it meant the operator would have to exert unreasonable force to rotate the tool. What to do? Test it!
The closer you can come to simulating the actual conditions of the application, the better your test data will be, but for most cases coefficients of friction can be determined using a simple tilt test from high school physics:
1) Obtain samples of the two materials in question, and make sure that in terms of surface finish, cleanliness, etc, that they are representative of the parts that will be used in the design.
2) Tilt until the sample slides, and record the angle. Repeat a number of times.
3) Use a little trigonometry and the simple friction equation to calculate the coefficient of friction.
A little engineering judgment is required to determine exactly how rigorous you need to be with your testing, but even the most rudimentary test is going to give you more confidence in your design than estimating from a bunch of contradictory numbers for ‘similar' materials that you dug up on the web. In my case, I spent about an hour testing, and came up with a value that was between the two extremes, but biased to the low side. I ended up having to make some design changes to improve the clamp force as well as decrease the normal force on the bushings in the unclamped state in order to reduce the torque required to rotate the tool.
Friction is an easy example to illustrate, but there are many other instances where experimentation makes sense, either to confirm theoretical values, prove out a concept, or provide data that is simply not otherwise available. Experimentation may also provide good qualitative information, or may highlight other aspects or issues that aren't apparent from calculation or CAD models. Tests and experiments are just another way we put our ‘dirty hands' philosophy to work, and our prototype shop gives us the tools to do so.
This article was authored by John Duffett. Mr. Duffett has been a mechanical engineer since 2005 with experience in analysis and design. He has experience and formal training in using machine tools, is a former bicycle mechanic, and likes to tinker with cars in his spare time. He usually has some dirt under his fingernails.
